Kelompok Besaran Di Bawah Ini Yang Merupakan Kelompok Besaran Turunan Adalah …
As I delve into the topic of kelompok besaran di bawah ini yang merupakan kelompok besaran turunan adalah …, it’s crucial to understand the concept of derived quantities in physics. Derived quantities are those that are 
derived from base quantities through multiplication or division, without any need for new units. They depend on fundamental units and mathematical operations.
When we talk about derived quantities Kelompok Besaran Di Bawah Ini Yang Merupakan Kelompok Besaran Turunan Adalah …, we’re referring to measurements like speed, acceleration, density, and many more. These quantities aren’t independent but rather calculated based on fundamental units such as length, mass, time, electric current, temperature, amount of substance, and luminous intensity.
In the realm of physics and mathematics, identifying which groups belong to derived quantities can enhance our understanding of how different physical parameters interrelate. By recognizing these relationships and dependencies among various measurements, we gain deeper insights into the underlying principles governing our universe.
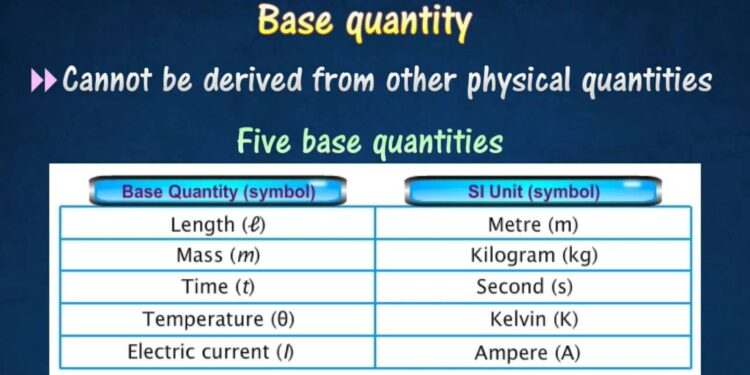
Understanding Base Quantities
As I delve into the realm of base quantities Kelompok Besaran Di Bawah Ini Yang Merupakan Kelompok Besaran Turunan Adalah …, it becomes apparent that these fundamental units form the building blocks for all other derived quantities. Imagine a world where everything we measure stems from these core values – length, mass, time, electric current, temperature, amount of substance, and luminous intensity. These base quantities serve as the foundation upon which the entire system of measurement stands.
When we grasp the essence of base quantities, we unlock a deeper understanding of how various physical properties are interrelated. For instance, length intertwines with area and volume; mass plays a pivotal role in 
determining force and density; time governs rates and frequencies. The interconnectedness of these base quantities underscores their significance in scientific calculations and everyday applications.
Exploring further into the concept reveals that each base quantity possesses its unique unit of measurement. Meters for length, seconds for time, kilograms for mass – these units provide us with standardized benchmarks to quantify our observations accurately. By adhering to established units tied to base quantities, scientists across disciplines ensure consistency and precision in their experimental findings.
Delving into the origins of base quantities unveils a rich history shaped by centuries of human curiosity and ingenuity. From ancient civilizations devising rudimentary measuring systems to modern-day advancements culminating in sophisticated metrological standards, the evolution of base quantities mirrors our relentless pursuit of knowledge and precision in quantifying the world around us.
Examples of Derived Quantities
When exploring the realm of derived quantities Kelompok Besaran Di Bawah Ini Yang Merupakan Kelompok Besaran Turunan Adalah …, it’s fascinating to delve into various examples that showcase how these quantities are formed and utilized in different fields. From simple equations to complex calculations, derived quantities play a crucial role in understanding the relationships between different physical parameters.
1. Speed and Velocity
One fundamental example of a derived quantity is speed, which is calculated by dividing the distance traveled by 
an object over time. On the other hand, velocity takes into account both speed and direction, making it a vector quantity. These concepts are essential in physics, mechanics, and everyday scenarios where motion is involved.
2. Acceleration
Acceleration is another derived quantity that represents the rate at which an object’s velocity changes over time. It can be positive (speeding up), negative (slowing down), or zero (constant velocity). Understanding acceleration is key in fields such as automotive engineering, sports science, and space exploration.
3. Force
Derived from mass and acceleration through Newton’s second law of motion, force is a vector quantity that describes the interaction between objects.
Exploring these examples sheds light on how derived quantities serve as building blocks for more advanced calculations and theories across different disciplines. By recognizing their significance and applications in real-world scenarios, we can appreciate the interconnectedness of physical phenomena and enhance our problem-solving skills.
